Bitcoin: Six Long Term Models

January 2022 update

This is not investment advice. Bitcoin is highly volatile. Past performance of back-tested models is no assurance of future performance. Only invest what you can afford to lose. You must decide how much of your investment capital you are willing to risk with Bitcoin. No warranties are expressed or implied. 

This article summarizes the status of six different models for Bitcoin’s price and market cap as of the beginning of January 2022. For details on the various models see my previous Substack articles or the YouTube presentation here. (This interview content represents my own views, not official views of OrionX or of CryptoScience.)

The models are:

1. A stock-to-flow model. This is expressed as a power law of market cap against the stock-to-flow, and there are 140 Block monthly data points. (There are 12 Block months, 4375 blocks each, per Block year of 52,500 blocks). With Bitcoin’s natural Block calendar basis, one year forward stock-to-flow is easily calculated.

2. An S-curve model. This market cap model uses a Weibull cumulative distribution function and is normalized to an ultimate $30 trillion market cap (intermediate between all gold and all global M2 money supply). The model is insensitive to the assumed market cap above $3 trillion at present, but higher ultimate market cap lengthens the characteristic time scale. Also 140 data points are included.

3. A “Lindy” power law model. This model has the price as a power law of the number of Block years elapsed, currently 13.66 Block years. 140 monthly data points.

4. A 48 Block month moving average floor model. This model is similar to a 200-week monthly average model and is exactly the length of a Halving cycle. 48 data points. The 48-month curve has acted as a floor under the price, throughout Bitcoin’s history.

5. A Bitcoin vs. Gold model. Here we look at the price of Bitcoin in terms of number of gold ounces, The bitcoin vs. gold price is an exponential function of the age of Bitcoin; this model uses calendar years with 9 years of monthly data.

6. An e-fold model. This model simply looks at how many factors of e (2.71828) the price has increased during one Block year.

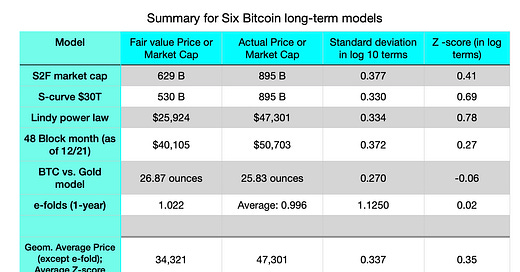
Table 1: Summary of the fair value price or market cap for six models, and the Z-score for each model. Actual prices and market cap are as of 1/2/22 except for the 48 Block month model from 12/8/21. For the 48 Block month model the fair value price is the 48-month average (a “floor price”) multiplied by a factor 10^(standard deviation in log10 price times the average Z score over the 48 months). Thus the effective Z-score shown in the last column is relative to the fair value determination. For the e-fold model we compare the number of factors of e price increase in the past year to the average trend value. (One e-fold is equivalent to the natural log of price increasing by one.). Market cap is converted to price using a present supply of 18.92 million Bitcoin.

The stock-to-flow model, (Lindy) power law model, and 48 month average floor model are based on others’ concepts. The S-curve, Bitcoin vs. gold exponential model, and the e-fold model are my own creations. All models give good fits and none can be rejected based on the data and statistical properties of the regressions.

For all models the actual price is close to or above the model fair value, but not by much in logarithmic terms. All models have Z-scores that are between -0.1 and 0.8, within a single standard deviation. Here Z is the number of standard deviations by which price or market cap is above the model price. In the case of the 48 Block month model, the Z score is relative to the fair value. Similarly, the Z-score for the e-fold model subtracts the average value.

The geometric average price of the first five models is about $34,300. There is certainly potential downside from the current price that would not destroy the validity of these models. Since standard deviations are all about 0.3 in log10 terms, that is a factor of 2, and any price less than $68,600, close to Bitcoin’s all time high, is not even one standard deviation removed from the average trend of the models.

The conclusion is: don’t worry too much about Bitcoin’s price moving by $5,000 or even $10,000. Zoom out, think logarithmically for long-term accumulation and savings purposes. Dollar cost averaging is your friend; all of these models have strong long term upward trends. For example, the Lindy power law model is on a 48% per annum trend, and the 48 Block month model is on a 99% per annum trend.

If you asked me which of the models are my favorite it would be those two. The Lindy power law model is a close approximation to the S-curve models at present. And the 48 Block month (or Gregorian calendar month) is easy to construct and has acted well as a price support floor, presumably since it is based on Bitcoin’s natural block reward Halving cycle.

Today’s price may be above average fair value by 38%, but it would only take several months of trend growth to overcome that difference.